Related Topics:
Math Worksheets
5.5 Net And Total Distanceap Calculus 14th Edition
Learn more about using nets to find the surface area of a polyhedron. After trying the questions, click on the buttons to view answers and explanations in text or video.
They're saying total distance the particle has traveled. So this is the total path length for the particle. So one way to think about it, you would integrate not the velocity function, if you integrate velocity, you get displacement, instead, you would integrate the speed function. The total area of all the polygons in the net, and hence the surface area of Polyhedron I, is 5(5 × 5) + 4(½ × 5 × 8) = 205 square units. To check your answers for the other polyhedra, the surface areas of the other polyhedra are provided below: A: 340 square units B: 408 square units C: 274 square units D: 300 square units. $ begingroup$ The 'net' total distance is actually displacement. You can just say you require the total distance, not the net total distance. And adding the word total to total displacement doesn't really make sense, since displacement is always a total, i.e last position - initial position. $ endgroup$ – john Jan 31 '18 at 12:55.
More Nets, More Surface Area
Let’s draw nets and find the surface area of polyhedra.
15.1 - Notice and Wonder: Wrapping Paper
Kiran is wrapping this box of sports cards as a present for a friend.
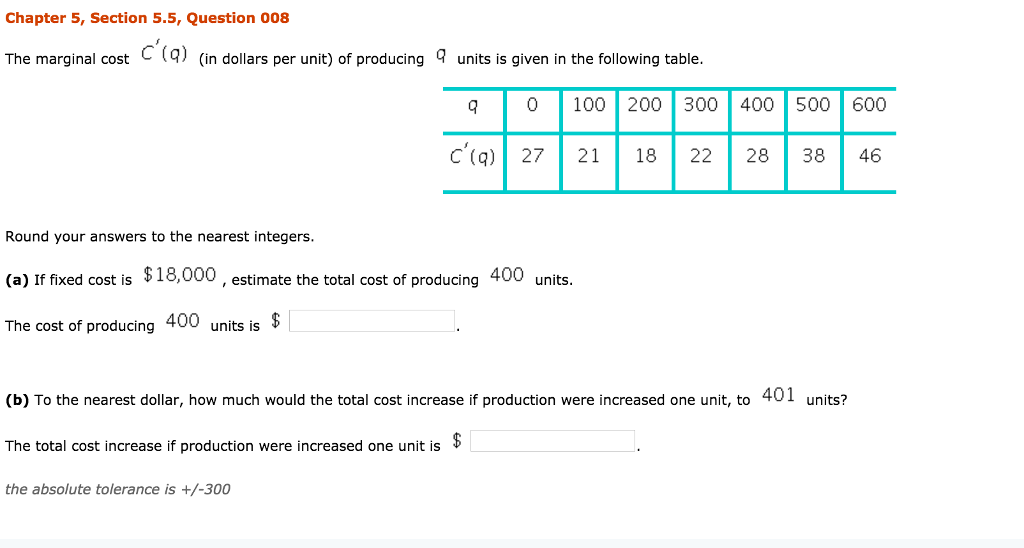
5.5 Net And Total Distanceap Calculus Ab
What do you notice? What do you wonder?
- See Possible Answers
The amount of wrapping paper needed is related to the surface area of the box.
The volume of the box determines what can fit inside.
There is not enough information to calculate either the surface area or the volume; the height of the box is missing.
We may estimate the height to be between 2.5 in and 4 in, since the height is clearly shorter than the 4 in side.If we had the height of the box, the surface area of the box could be calculated by finding the area of all of the faces, while the volume of the box could be calculated by multiplying the depth, width, and height.
Other observations are also possible.
- See Video 2 for Whole Lesson
15.2 - Building Prisms and Pyramids
Your teacher will give you one of the following drawings of a polyhedron. You will draw its net and calculate its surface area.
- Show Image
1. What polyhedron do you have?
2. Study your polyhedron. Then, draw its net on graph paper. Use the side length of a grid square as the unit.
3. Label each polygon on the net with a name or number.
4. Find the surface area of your polyhedron. Show your thinking in an organized manner so that it can be followed by others.
- Hints
You may label faces of the polyhedron drawing, to make sure that you draw adjacent faces as adjacent in your net.
Your net must be drawn to scale. Use the grid squares of the graph paper as units of measurement.
Drawing a net from a drawing of a polyhedron takes practice. You may need a few drafts.
- See Possible Answers
This is a complete solution for Polyhedron I. The reasoning is similar for all the polyhedra.
1. Polyhedron I is a composite of a square pyramid and a cube.
2-3.
4. Faces A-D have the same area and have been shaded the same color. Faces E-I also have the same area as each other.
The total area of all the polygons in the net, and hence the surface area of Polyhedron I, is 5(5 × 5) + 4(½ × 5 × 8) = 205 square units.To check your answers for the other polyhedra, the surface areas of the other polyhedra are provided below:
A: 340 square units
B: 408 square units
C: 274 square units
D: 300 square units
E: 216 square units (The triangular bases are isoceles triangles.)
F: 240 square units
G: 156 square units
H: 256 square units
15.3 - Comparing Boxes
Here are the nets of three cardboard boxes that are all rectangular prisms. The boxes will be packed with 1-centimeter cubes. All lengths are in centimeters.
1. Compare the surface areas of the boxes. Which box will use the least cardboard? Show your reasoning.
2. Now compare the volumes of these boxes in cubic centimeters. Which box will hold the most 1-centimeter cubes? Show your reasoning.
- Answers
1. Surface area of A: 2(3 × 3) + 4(2 × 3) = 42 square cm
Surface area of B: 2(3 × 6) + 2(3) + 2(6) = 54 square cm
Surface area of C: 6(3 × 3) = 54 square cm
A will use the least cardboard, as it has the least surface area.2. Volume of A: 3 × 3 × 2 = 18 cubic cm
Volume of B: 3 × 6 × 1 = 18 cubic cm
Volume of C: 3 × 3 × 3 = 27 cubic cm
C will hold the most 1-centimeter cubes (27 cubes), as it has the largest volume.
Figure C shows a net of a cube. Draw a different net of a cube. Draw another one. And then another one. How many different nets can be drawn and assembled into a cube?

Lesson 15 Summary
The surface area of a polyhedron is the sum of the areas of all of the faces.
Because a net shows us all faces of a polyhedron at once, it can help us find the surface area. We can find the areas of all polygons in the net and add them.
- Show Image
A square pyramid has a square and four triangles for its faces. Its surface area is the sum of the areas of the square base and the four triangular faces: (6 × 6) + 4 × (½ × 5 × 6) = 96 square units.
The surface area of this square pyramid is 96 square units.
Practice Problems
1. Jada drew a net for a polyhedron and calculated its surface area.
a. What polyhedron can be assembled from this net?
b. Jada made some mistakes in her area calculation. What were the mistakes?
c. Find the surface area of the polyhedron. Show your reasoning.
- Answers
a. This net can be assembled into a triangular prism (with right triangular bases).
b. The individual triangles have an area of 6 sq cm, not 12 sq cm.
c. 20 sq cm + 16 sq cm + 12 sq cm + 2(6 sq cm) = 60 sq cm.

2. A cereal box is 8 inches by 2 inches by 12 inches. What is its surface area? Show your reasoning. If you get stuck, consider drawing a sketch of the box or its net and labeling the edges with their measurements.
- Answers
The surface area of this cereal box is 2(8 × 12) + 2(2 × 12) + 2(2 × 8) = 272 square units.
Other strategies are also possible.
3. Twelve cubes are stacked to make this figure.
a. What is its surface area?
b. How would the surface area change if the top two cubes are removed?
- Answers
a. Consider 1 face of 1 cube to equal 1 square unit.
The green face is 6 square units. It is congruent with another face which is obscured from view.
The back face, obscured from view, is 6 square units.
The blue faces total 6 square units, and the yellow faces also total 6 square units.
The surface area of this figure is (6 × 2) + 6 + 6 + 6 = 30 square units.b. If the top two cubes were removed, the green face and its opposing face would be 5 square units each.
The back face would be 4 square units.
There would still be 6 blue faces (facing the top of the figure).
There would be 4 yellow faces.
The surface area of the new figure would be (5 × 2) + 4 + 6 + 4 = 24 square units.
4. Here are two polyhedra and their nets. Label all edges in the net with the correct lengths.
- Show Image
5. a. What three-dimensional figure can be assembled from the net?
b. What is the surface area of the figure? (One grid square is 1 square unit.)
- Show Image
- Answers
a. This net can be assembled into a square pyramid.
b. The surface area of a polyhedron can be found from the total area of all the polygons in its net.
The area of the central square is 4 × 4 = 16 square units.
The area of the four triangles is 4 × ½ × 4 × 5 = 40 square units.
The Open Up Resources math curriculum is free to download from the Open Up Resources website and is also available from Illustrative Mathematics.
Try the free Mathway calculator and problem solver below to practice various math topics. Try the given examples, or type in your own problem and check your answer with the step-by-step explanations.
We welcome your feedback, comments and questions about this site or page. Please submit your feedback or enquiries via our Feedback page.
Answer
Work Step by Step
